
The pitch channel is autonomous, it can be regarded independently of
the other two channels movement. The roll and yaw channels are closely
connected. This is explained by the presence of angular velocity of the
orbital coordinate system in the orbit plane, which leads to the appearance
of gyroscopic cross connections:
J
x
dω
x
dt
+ (
J
z
−
J
y
)Ω
ω
y
=
M
x
;
J
y
dω
y
dt
+ (
J
z
−
J
x
)Ω
ω
x
=
M
y
;
J
z
dω
z
dt
=
M
z
.
(10)
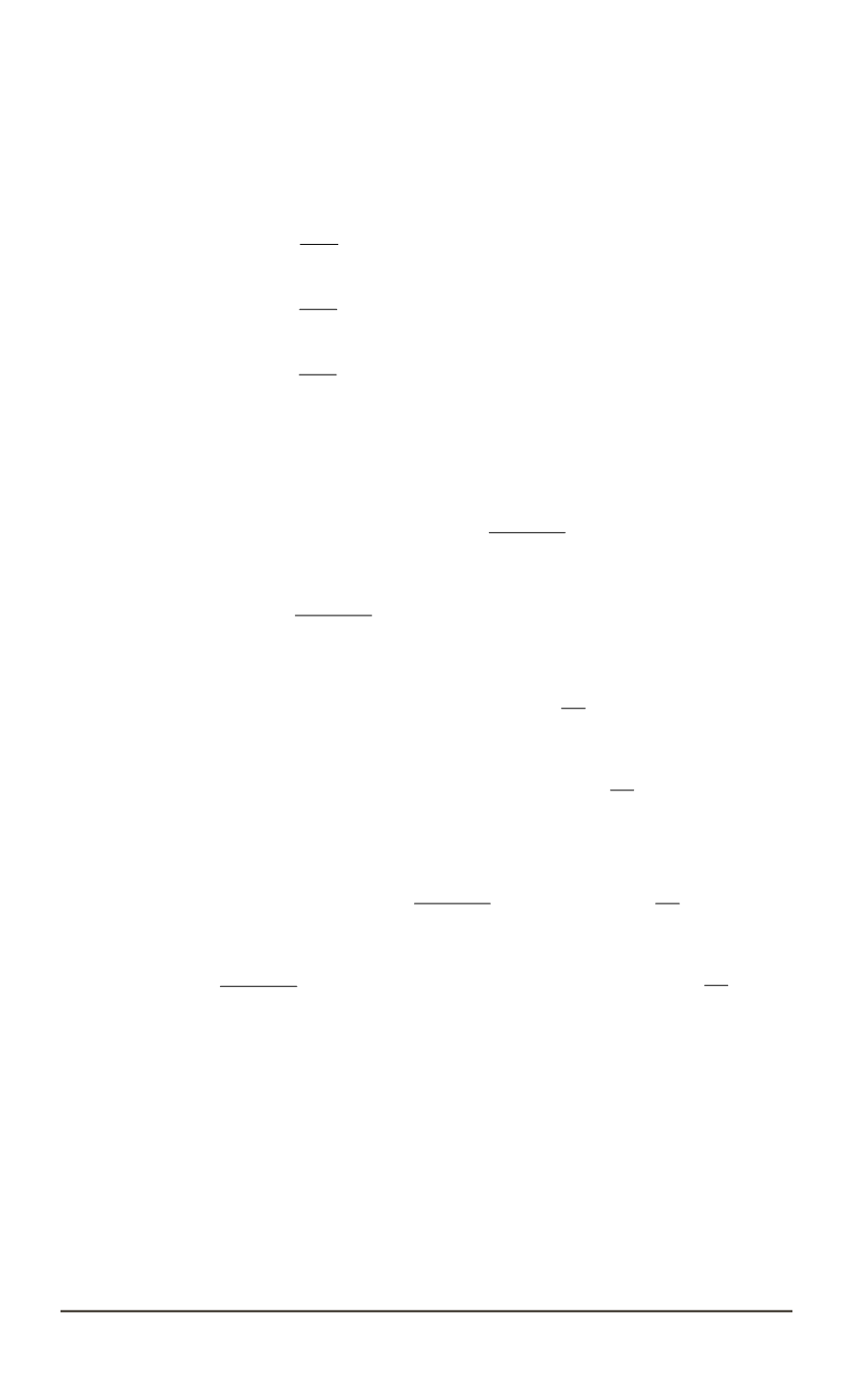
Using systems (8)–(10) for the connected channels, we obtain:
˙
γ
˙
ω
x
˙
ψ
˙
ω
y
=
0
1
−
Ω 0
0
0
0
−
J
z
−
J
y
J
x
Ω
Ω 0
0
1
0
−
J
z
−
J
x
J
y
Ω 0
0
×
×
γ
ω
x
ψ
ω
y
+
0 0
1
J
x
0
0 0
0
1
J
y
M
x
M
y
;
A
=
0
1
−
Ω 0
0
0
0
−
J
z
−
J
y
J
x
Ω
Ω 0
0
1
0
−
J
z
−
J
x
J
y
Ω 0
0
;
B
=
0 0
1
J
x
0
0 0
0
1
J
y
;
(11)
C
= 1 0 0 0
.
(12)
In accordance with (2) and on the basis of (11) and (12) for the zero
level of a discrete system with
h
tract let’s put
A
0
=
A
T
=
1 0
a
31
h
0
h
1 0
a
42
h
a
31
h
0 1 0
0
a
24
h h
1
;
(13)
ISSN 0236-3933. HERALD of the BMSTU. Series “Instrument Engineering”. 2014. No. 5
7

















