
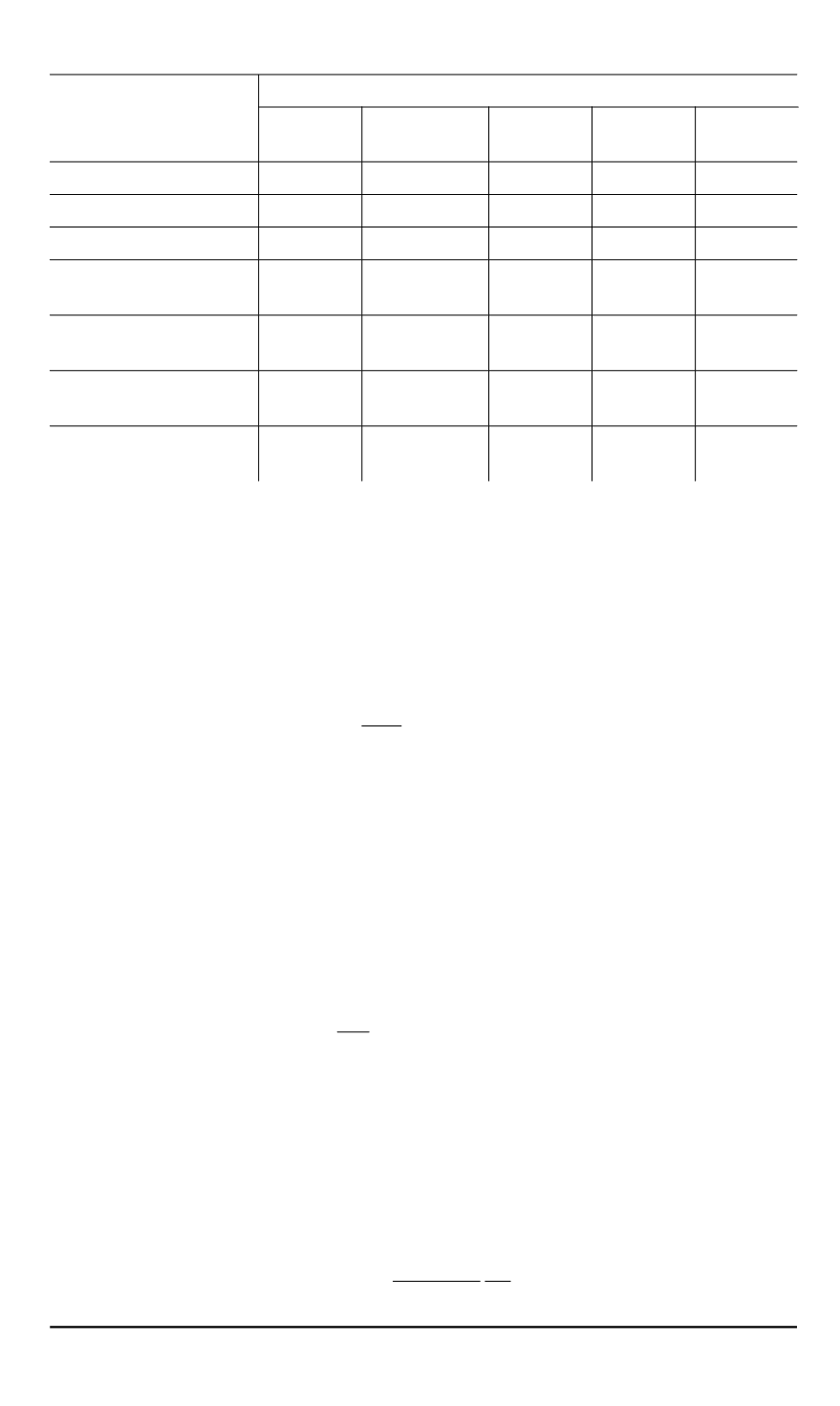
Physical parameters of materials
Material parameters
Material
Aluminum
(Д16Т)
Steel
(12Х18Н10Т)
Silicon (Si) Sapphire
(Al
2
O
3
)
Quartz
(SiO
2
)
Density,
ρ
, kg/m
3
2800
7900
2320
3980
2220
Poisson’s ratio,
ν
0.33
0.30
0.28
0.25
0.18
Young’s modulus,
E
, Па 7.08
∙
10
10
1.98
∙
10
11
1.30
∙
10
11
4.40
∙
10
11
7.20
∙
10
10
Heat expansion rate,
α
,
1/
◦
C
2.30
∙
10
−
5
1.66
∙
10
−
5
4.20
∙
10
−
6
6.60
∙
10
−
6
6.00
∙
10
−
7
Specific heat,
C
p
,
J/kg
∙
◦
C
922
462
1414
790
728
Volumetric heat capacity,
С
V
, J/m
3
∙
◦
C
2.58
∙
10
−
6
3,65
∙
10
−
6
3.28
∙
10
−
6
3.14
∙
10
−
6
1.62
∙
10
−
6
Heat conductivity
coefficient,
k
, W/(m
∙
◦
C)
237
15
150
40
1,35
Stress tensors and deformation tensors consist of
x
,
y
and
z
normal
components and
x–y
,
y–z
и
z–x
tangent components.
The equations of elastic medium motion are obtained if the force of
internal stresses
∇ ∙
σ
is equated to the product of acceleration and mass
of a solid body volume unit (i.e. its density)
ρ
¨u
. The vector form of the
motion equation is
ρ
∂
2
u
∂t
2
=
∇ ∙
σ.
(5)
Here
ρ
— volume density;
u
— displacement vector.
Equations (4) and (5) form a total system of differential equations
in partial derivatives for stresses and deformations. Boundary conditions
should be added to (4) и (5), but we will not dwell on them.
The connection of deformation with temperature is set using thermodynaics
laws. The equation of thermal conductivity at a small thermal disturbance
(i.e. with
(
T
−
T
0
)
/T
0
1
) can be written as
C
V
∂T
∂t
− ∇
(
k
∇
T
) = ˙
q,
(6)
where
C
V
=
ρC
p
— volumetric heat capacity;
C
p
— specific heat;
k
(
χ
)
—
heat conductivity coefficient;
˙
q
— heat source, namely, heat generation rate
in the unit of volume.
In case of thermoelastic heating, the heat source for an isotropic material
is determined as follows:
˙
q
=
−
EαT
0
(1
−
2
ν
)
∂e
∂t
,
(7)
ISSN 0236-3933. HERALD of the BMSTU. Series Instrument Engineering. 2015. No. 2
31
















